
Pedal Triangles
by:
Brandt Hacker
For this assignment we are going to look at pedal triangles and how moving the pedal point effects the pedal triangle. First we must define what a pedal point is. A pedal triangle is created when we take a point and draw three perpendicular lines to an existing triangle. If we use the intersection of these perpendicular lines with the sides of the original triangle to create three points, we have the three points that create the pedal triangle. Below is an example of two pedal triangles, one inside and one outside.
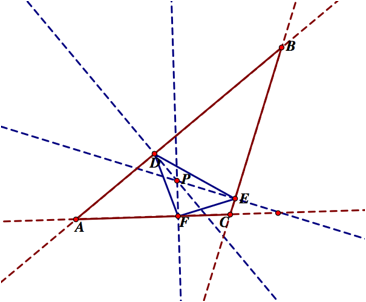
Above, the pedal point, point P, is located inside triangle ABC and the pedal triangle DEF is also located within.

Above we see just one example of a pedal point located outside the circle. This particular example illustrates that it is possible for a pedal triangle to be located completely outside the original triangle, though the pedal point being outside does not necessitate this.
Next we will look at what happens when the pedal point is located at various centers of the triangle. We will look at the incenter, orthocenter, centroid, and circumcenter as pedal points of triangle ABC.
First we look at what happens when the pedal point is located at the incenter. For this image and the ones that follow, the perpendicular lines from the pedal point have been removed.
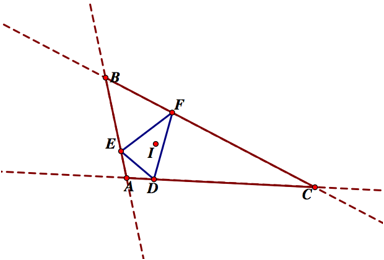
Due to the fact that a triangle’s incenter is always located inside the triangle, when we make the pedal point the incenter, the resulting pedal triangle will always be located within the triangle.
Next we will take a look at what happens when the pedal point is the centroid of the triangle.
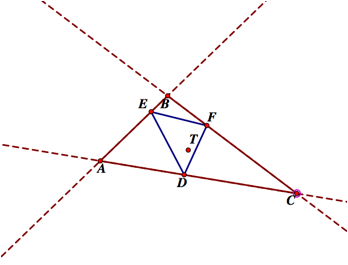
Here the pedal point and centroid are the same point, point T. Just like the incenter, the centroid is always located within the triangle. Therefore, when the pedal point is at the centroid the resulting triangle will always be located within the original triangle.
Next we will look at the other two centers. Due to the fact that a triangles orthocenter can be inside, outside, or on the triangle, we have several different situations to consider.
Orthocenter outside triangle ABC…
Here we see that the pedal triangle can lie partially inside and outside it’s parent triangle.
Orthocenter on triangle ABC…
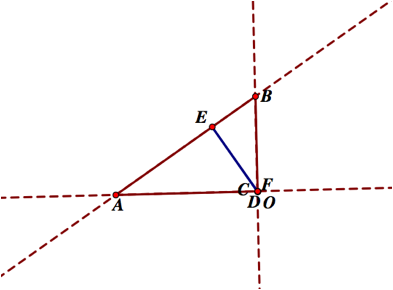
The orthocenter lies on a triangle when the triangle is a right triangle. If it is obtuse it lies on the outside, and if it is acute it lies within the triangle. Here we see that points C, D, F, and O are concurrent in a right triangle. Because of this, the “pedal triangle” is not actually a triangle at all, but rather a line segment.
Lastly, the orthocenter inside of triangle ABC…
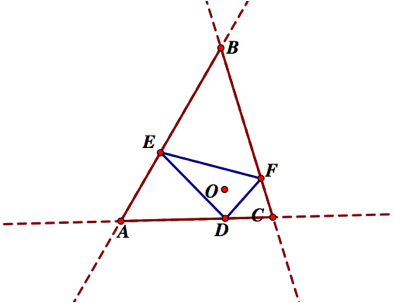
When the orthocenter is located inside a triangle, the pedal triangle created from said orthocenter has a very special property. The triangle pictured in blue is the triangle with the minimum area that is inscribed by triangle ABC; this is called the orthic triangle.
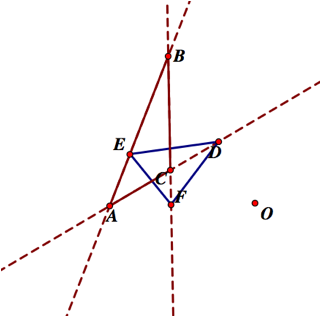
Next we will look at what happens when the pedal point is the same as the circumcenter of triangle ABC.
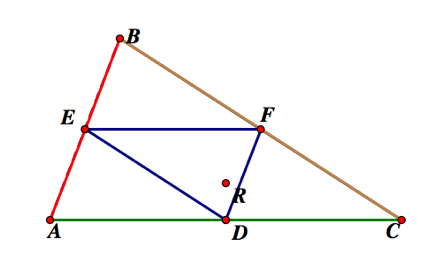
Circumcenter outside triangle ABC….
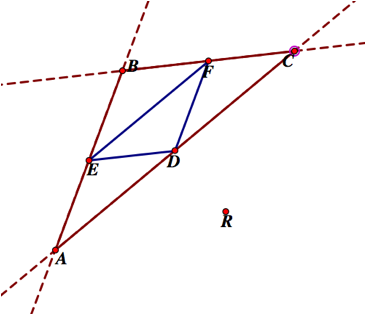
Here we see that even though the circumcenter, point R, is located outside the circle, the pedal triangle from this pedal point is still located inside the triangle.
Circumcenter/pedal point on triangle ABC…
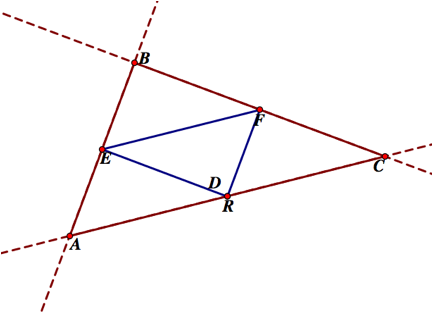
Lastly, what if the circumcenter/pedal point lies inside the triangle…

If we look at the three diagrams above, we see that there are actually 5 triangles that can be seen after creating the pedal triangle. Using the image above, we see triangle ABC, EFD, AED, BEF, and DFC. What’s interesting about these triangles is that all 5 are similar to one another and excluding triangle ABC, they are all congruent to one another. Lets examine why this is…
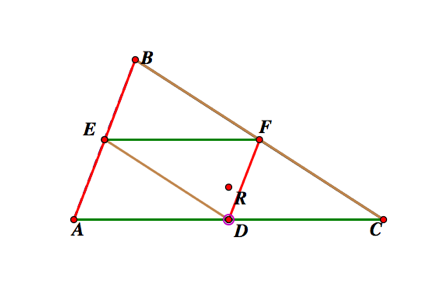
Because R is the circumcenter of triangle ABC the perpendicular lines from R are all midpoints of the segments that they intersect. In other words, point D, F, and E are all midpoints of their respective segments. After determining that each of the vertices of our pedal triangle is a midpoint we show the congruence of segments from this point forward by color. If two colors are the same, then those segments are congruent.
In the image above we see that ![]() ,
, ![]() , and
, and ![]() .
.
After determining these ocngruent segments we are one step closer to showing the similarity between triangle ABC and the other 4 triangles pictured above. We see that angle B is congruent to itself in triangles EBF and ABC. Along with this we see that triangle EBF and ABC are similar by SAS. Because AB is twice EB and BC is twice, the proportions also hold s for the third side; AC is twice EF. If we continue with this for the other angles of triangle ABC we end up with the following image:
From this image we see that all of the triangle ![]() by
side-side-side.
by
side-side-side.